Heat escapes (or transfers) from inside to outside (high temperature to low temperature) by three mechanisms (either individually or in combination) from a home:

• Conduction
• Convection
• Radiation

Examples of Heat Transfer by Conduction, Convection, and Radiation

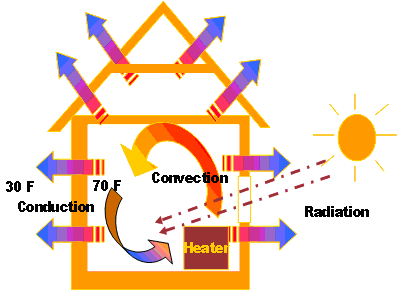
Click here to open a text description of the examples of heat transfer by conduction, convection, and radiation

• Conduction: heat moving through walls of a home from high temperature inside to low temperature outside.
• Convection: heat circulating within the rooms of a house.
• Radiation: Heat from the sun entering a home.

Credit: © Penn State is licensed under CC BY-NC-SA 4.0 [1]

Conduction

Conduction is a process by which heat is transferred from the hot area of a solid object to the cool area of a solid object by the collisions of particles.

In other words, in solids the atoms or molecules do not have the freedom to move, as liquids or gases do, so the energy is stored in the vibration of atoms. An atom or molecule with more energy transfers energy to an adjacent atom or molecule by physical contact or collision.

In the image below, heat (energy) is conducted from the end of the rod in the candle flame further down to the cooler end of the rod as the vibrations of one molecule are passed to the next; however, there is no movement of energetic atoms or molecules.

Click the play button to start the animation.

With regard to residential heating, the heat is transferred by conduction through solids like walls, floors, and the roof.

Click the play button to start the animation.

Convection

Convection is a process by which heat is transferred from one part of a fluid (liquid or gas) to another by the bulk movement of the fluid itself. Hot regions of a fluid or gas are less dense than cooler regions, so they tend to rise. As the warmer fluids rise, they are replaced by cooler fluid or gases from above.

In the example below, heat (energy) coming from candle flame rises and is replaced by the cool air surrounding it.

Click the play button to start the animation.

In residential heating, convection is the mechanism by which heat is lost by warm air leaking to the outside when the doors are opened, or cold air leaking into the house through the cracks or openings in walls, windows, or doors. When cold air comes in contact with the heater in a room, it absorbs the heat and rises. Cold air, being heavy, sinks to the floor and gets heated, and thus slowly heats the whole room air.

Instructions: Press the play button below and observe what happens to the cold air (blue arrows) as it enters the house and encounters the warm air (red arrows) coming from the heating vent:

Radiation

Radiation is the transfer of heat through electromagnetic waves through space. Unlike convection or conduction, where energy from gases, liquids, and solids is transferred by the molecules with or without their physical movement, radiation does not need any medium (molecules or atoms). Energy can be transferred by radiation even in a vacuum.

In the image below, sunlight travels to the earth through space, where there are no gases, solids, or liquids.

Click the play button to start the animation.

Test Yourself

First, identify the type of home heat loss pictured in images A-J as either: conduction, convection or radiation. Then click and drag each image down to the correct category at the bottom of the screen.

Reducing Energy Consumption

There are two ways in which we can reduce energy consumption.

1. The most cost-effective way is to improve the home’s “envelope”—the walls, windows, doors, roof, and floors that enclose the home—by improving the insulation (conduction losses) and sealing the air leaks with caulking (convection losses).
2. The second way to reduce the energy consumption is by improving the efficiency of the furnace that provides the heat.

Conduction and Convection

Click here to open a text description of the Conduction and Convection diagram

Line drawing of a house with arrows pointing out from the walls and roof showing conduction & arrows flowing in a circular motion inside the house showing convection.

Credit: © Penn State is licensed under CC BY-NC-SA 4.0 [1]

Main Factors of Heat Loss

What does a house's heat loss depend on? Complete the activity below to find out the three main factors leading to heat loss.

Most heat is lost through a house's walls through conduction. As you learned from the activity on the previous screen, the amount of heat loss depends on three factors:

• Size of the house (area through which the heat can escape)
• Local weather or climatic conditions:
  • The inside temperature is often constant at a comfortable temperature of 65°F.
  • As the outside temperature falls lower than 65°F, the heat is lost to the outside.
  • The higher the temperature difference, the higher the heat loss to outside.
  • By calculating the Heating Degree Days (HDD), we can determine how many degrees the mean temperature fell below 65ºF for the day.
• Wall's capacity to resist heat loss.
  • Insulation is rated in terms of thermal resistance, called R-value, which indicates the resistance to heat flow.
  • The higher the R-value, the greater is the insulating effectiveness.

Local weather or climatic conditions are one of three factors that affects the amount of heat loss through conduction. When examining weather conditions, we look at both the inside and outside temperature of a home.

The inside temperature is usually taken as a standard comfort temperature of 65ºF. The outside temperature varies by the hour. Knowing this information can help us to understand two concepts:

• Average outside temperature = Average of the maximum and minimum temperature during the day
• Heating Degree Day (HDD) = The temperature difference through which air has to be treated, or how many degrees the mean temperature fell below 65ºF for the day. It is also an index of fuel consumption.

Temperatures Inside and Outside a House

Credit: © Penn State is licensed under CC BY-NC-SA 4.0 [1]

Determining Heating Degree Day (HDD)

The formula for determining the Heating Degree Day (HHD) is:

$${\text{HDD = T}}_{\text{base}}-{\text{T}}_{\text{a}}$$

To calculate HDD:

1. Determine the base temperature or inside temperature: $${\text{T}}_{\text{base}}\text{ = usually 65}°\text{F}$$
2. Find the day's average outside temperature using this formula: $${\text{T}}_{\text{a}}\text{ = average outside temperature = }\frac{{\text{T}}_{\text{max}}+{\text{T}}_{\text{min}}}{\text{2}}$$
3. Use the HDD formula to solve:
   $${\text{T}}_{\text{base}}{\text{ - T}}_{\text{a}}$$

Try This!

Click the play button below, and observe the temperature changes. Then calculate the average temperature and the Heating Degree Day.

Heating Degree Day

For this problem, we need to calculate HDD for one full week. The data that is given is each day, what is the outside temperature -- average outside temperature. For example, Sunday, the average outside temperature is 49 degrees F. Monday 47 degrees Fahrenheit, Tuesday it’s 51 and Wednesday it is 60 degrees F and on Thursday it is 65, Friday, 67 and on Saturday it is 58.

So we need to calculate heating degree days (HDD) for each day. One day, that is Sunday, the outside temperature is 65 minus 49 the outside temperature will give you the degree days. Similarly, 65 minus 47° Fahrenheit, one day times 65 minus 51 in this case. One day times 65 minus 60 and one day 65 minus 65. This would be zero. One day 65 minus 67. Remember when it exceeds 65, the heating degree days would be zero. In this case, also it is zero. One day on Saturday it is 65 minus 58.

This case it is 7.

These two are zeros, this is 5 and this is 14 and this happens to be 18 and here it is 16. So the total sum is for one full week is 60 degree days.

This is equal to 60 degree days.

In previous examples, we are assuming that the outside temperature remains the same for all 150 heating days in a season. This is not realistic, but it explains the method to calculate the HDD. In a more realistic example, we need to find the temperature difference for each day and add all the temperature differences.

We will now look at Seasonal Heating Degree Days (HDD), which is the sum of temperature differences of ALL days - rather than just 1 day or 1 week - during which heating is required.

The table below provides Seasonal HDDs for selected places in the United States. The higher HDD indicates a higher heat loss and therefore, higher fuel requirements.

HDD is used to estimate the amount of energy required for residential space heating during a cool season, and the data are published in local newspapers or on the National Weather Service website.

Source: NOAA [2]

Calculating Seasonal Heating Degree Days

To calculate Seasonal Heating Degree Days, use this formula:

Remember, in months where the average temperature is equal to or greater than 65, there will be no heating degree days, so the value for the month will be 0.

Example 1

Given the following set of average temperatures, by month, for State College, PA, calculate the HDD for the heating season:

Please watch the following (2:32) presentation about problem #1:

Example 2

If Ms. S. Belle moves from Birmingham, AL (HDD=2,800) to State College, PA (HDD=6,000) how much can she expect her heating bill to increase?

Please watch the following 1:34 presentation about problem #2:

As we have learned, most heat is lost through a house's walls through conduction. One of the three factors that affect heat loss is a wall's capacity to resist heat loss.

We will now look at how to calculate the rate of heat loss of the walls of a house, using the following formula:

From the above equation, it can be seen that once the house is built, these two variables will NOT change:

• The area of the walls
• The R-value of the walls

The only variable that will change is the temperature difference between inside and outside.

Example

Calculate the heat loss for a 10 ft by 8 ft wall, insulated to R-value 22. The inside temperature is maintained at 70° F. The temperature outside is 43° F.

Please watch the following 2:25 presentation about Hourly Heat Loss:

Now that you know how to calculate hourly heat loss, how would you calculate daily heat loss?

Since there are 24 hours in a day, you would simply multiply the hourly heat loss by 24.

Example

What is the hourly and daily heat loss of a 15-ft by 15-ft room with an 8-ft ceiling, with all surfaces insulated to R13, with inside temperature 65°F and outside temperature 25°F?

$$HourlyHeatLossrate=\frac{Q(BTUs)}{t(hour)}=\frac{(480f{t}^2\times \left({65}^{o}F-{25}^{o}F\right)}{13\frac{f{t}^2{}^{o}Fh}{BTU}}=1,477\frac{BTUs}{h}$$

Once we know the heat loss rate per hour, we can determine the heat loss per day by multiplying by 24 (hours in a day).

In a 24-hour period or one day, the heat loss would be:

$$DailyHeatLossperday=\frac{1477BTUs}{h}x\frac{24h}{day}=35,448\frac{BTUs}{day}$$

$$HeatLoss\left(\frac{BTUs}{h}\right)=\frac{Area(f{t}^2)\times TemperatureDifference{(}^{o}F)}{Rvalue\left(\frac{f{t}^2{}^{o}Fh}{BTU}\right)}$$

Area (ft²) is the sum of the area of all four walls. Each wall is 8' x 15', or 120 sq. feet, so take 4 x 120 sq ft to get 480 ft² in the equation.

In the previous calculations, we determined hourly and daily heat loss. How do we calculate annual or seasonal heat loss?

Since the temperature outside the house may not remain the same day after day, the heat loss will vary by the day. Thus, to obtain the heat loss for a whole year, we do the following:

• Calculate heat loss for each day.
• Add the heat loss for all days in a year that needed heating.
• Leave the R-value of the wall and the area of the wall the same – they will not change.
• Determine the difference between inside and outside temperature, since it will change for each day.

Recall that the formula for daily heat loss is:

Thus, theoretically, we would need to perform this calculation for every day of the 365-day calendar year.

For example, if the average outside temperature were to be 35°F, 32°F, 28°F, and so on for each day, the heat loss for the whole year or the season can be calculated as follows:

$$\begin{array}{l}\text{Heat Loss = }\underset{\text{day 1 heat loss}}{\underbrace{\frac{480f{t}^2\times \left(65-\stackrel{\text{outside temp for day 1}}{\stackrel{︸}{35}}\right)\text{ °F}}{13\frac{f{t}^2\text{ °F h}}{\text{BTU}}}\times 24\frac{h}{day}}}+\underset{\text{day 2 heat loss}}{\underbrace{\frac{480f{t}^2\times \left(65-\stackrel{\text{outside temp for day 2}}{\stackrel{︸}{32}}\right)\text{ °F}}{13\frac{f{t}^2\text{ °F h}}{\text{BTU}}}\times 24\frac{h}{day}}}+\\ \underset{\text{day 3 heat loss}}{\underbrace{\frac{480f{t}^2\times \left(65-\stackrel{\text{outside temp for day 3}}{\stackrel{︸}{28}}\right)\text{ °F}}{13\frac{f{t}^2\text{ °F h}}{\text{BTU}}}\times 24\frac{h}{day}}}+\mathrm{...}\text{ and so on for all heating days}\text{.}\end{array}$$

Since the area (480 ft²), R-value $\frac{f{t}^2°FBTU}{h}$,and 24 h in a day are common for ALL heating days, we can bring those out and rewrite the equation as:

$$\begin{array}{l}=\frac{480f{t}^{2}x}{13\frac{f{t}^2{}^{o}Fh}{BTU}}\times 24\frac{h}{day}\left\{{\left(65-35\right)}^{o}F+{\left(65-32\right)}^{o}F+{\left(65-28\right)}^{o}F\mathrm{...}soonforallheatingdays\right\}\\ \\ =\frac{480f{t}^{2}x}{13\frac{f{t}^2{}^{o}Fh}{BTU}}\times 24\frac{h}{day}\left\{{30}^{o}F+{33}^{o}F+{37}^{o}F+soonforallheatingdays\right\}\\ \\ Where\left\{{30}^{o}F+{33}^{o}F+{37}^{o}F+soonforallheatingdays\right\}isthesumofHeatingDegreeDays\end{array}$$

This equation can be even further simplified. The formula for Annual or Seasonal heat loss can be written in general terms as:

$$HeatLossinaSeason=\frac{Area(f{t}^2)}{Rvalue\left(\frac{f{t}^2{}^{o}Fh}{BTU}\right)}\times 24\frac{h}{day}\times (HDDfortheseason)$$

Example 1

Please watch the following 3:29 presentation on Example Problem #1. For the 150-day heating season in Roanoke, VA, the average temperature was 47° F. How much heat is lost through a 176 ft² wall (R=16) during the entire season?

Example 2

Please watch the following 3:42 presentation on Example Problem #2. In Fargo, ND, the heating season lasts about 220 days and the average outside temperature is around 27° F. How much heat is lost through an 8 ft by 6 ft window (R=1) during the heating season?