Trilateration is an alternative to triangulation that relies upon distance measurements only. Electronic distance measurement technologies make trilateration a cost-effective positioning technique for control surveys. Not only is it used by land surveyors, trilateration is also used to determine location coordinates with Global Positioning System satellites and receivers.
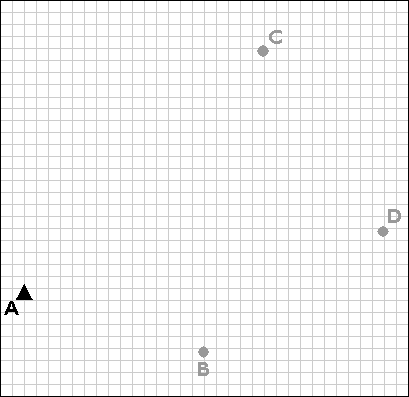
Trilateration is used to extend control networks by establishing new control points (B, C, and D) based upon existing control points (A).
Trilateration networks commence the same way as triangulation nets. If only one existing control point is available, a second point (B) is established by open traverse. Using a total station equipped with an electronic distance measurement device, the survey team measures the azimuth α and baseline distance AB. The total station operator may set up her instrument over point A, while her assistant holds a reflector mounted on a shoulder-high pole as steadily as he can over point B. Depending on the requirements of the control survey, the accuracy of the calculated position B may be confirmed by astronomical observation.
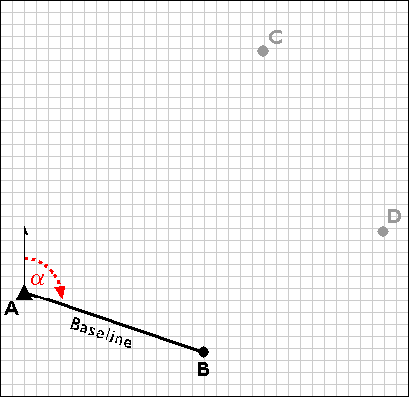
Establishing a second control point (B) in a trilateration network.
Next, the survey team uses the electronic distance measurement feature of the total station to measure the distances AC and BC. Both forward and backward measurements are taken. After the measurements are reduced from slope distances to horizontal distances, the law of cosines can be employed to calculate interior angles, and the coordinates of position C can be fixed. The accuracy of the fix is then checked by plotting triangle ABC and evaluating the error of closure.
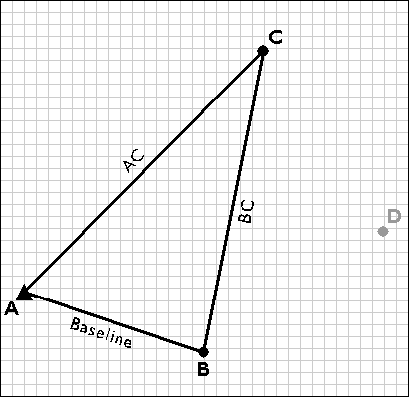
Measuring the distances AC and BC.
Next, the trilateration network is extended by measuring the distances CD and BD, and fixing point D in a plane coordinate system.
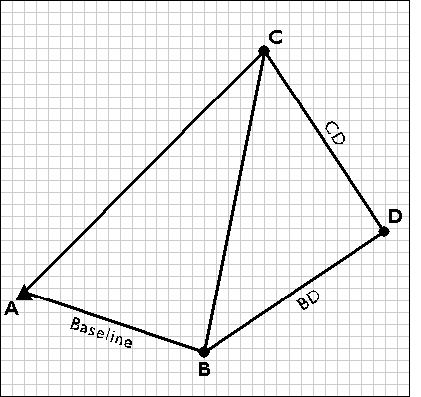
Fixing control point D by trilateration.
| Try This! |
Use trilateration to determine a control point locationTrilateration is a technique land surveyors use to calculate an undetermined position in a plane coordinate system by measuring distances from two known positions. As you will see later in this chapter, trilateration is also the technique that GPS receivers use to calculate their positions on the Earth's surface, relative to the positions of three or more satellite transmitters. The purpose of this exercise is to make sure you understand how trilateration works. (Estimated time to complete: 5 minutes.) Note: You will need to have the Adobe Flash player installed in order to complete this exercise. If you do not already have the Flash player, you can download it for free from Adobe.
Once you have finished viewing the grid, close the popup window. |
| Practice Quiz | Registered Penn State students should return now to the Chapter 5 folder in ANGEL (via the Resources menu to the left) to take a self-assessment quiz about Horizontal Positions. You may take practice quizzes as many times as you wish. They are not scored and do not affect your grade in any way. |
 |
Students who register for this Penn State course gain access to assignments and instructor feedback, and earn academic credit. Information about Penn State's Online Geospatial Education programs is available at http://gis.e-education.psu.edu. |