Carving the Canyon, and More on Radioactive Dating, and Radiocarbon ages of "Old" Living Clams
Many people are interested in the carving of the Canyon, and the age of the Earth, and related topics. Often, this interest is linked to certain objections to the science of an old Earth, possibly arising from the deeply mistaken idea that a person cannot be a good member of some religions while accepting the science of geology. (Full disclosure: Dr. Alley is a long-time member of a reconciling Methodist church.)
The short essays below address a few of the questions that Dr. Alley has heard in these areas, and may serve as starting points if you have additional questions.
Carving the Canyon
Really big, deep canyons are often found closer to mountain ranges than the Grand Canyon is—it’s fairly easy to cut deeply into something really high, while the river doing the cutting is still high and steep. So why is that immense canyon out there in Arizona, and how long did it take to cut?
A vigorous river is capable of cutting downward at 1 mm/year (or more, and glaciers may cut faster than that). At 1 mm/year, it takes 25 years to cut an inch, or only about 1.6 million years to cut a mile down and make the Grand Canyon. Usually, rivers don’t cut as fast as 1 mm/year because the rivers quickly get down close to sea level, which makes the river’s slope smaller and slows the erosion. But, the Grand Canyon probably took longer than that, as we’ll see soon, in part because the river had to cut several times deeper than the Canyon is!
The Grand Canyon likely owes its existence to several events, including opening of the Gulf of California causing “river piracy”, stealing a different river to run through the Canyon. As we saw way back in Module 2, sea-floor spreading began in the Gulf of California about 5 million years ago, and this likely triggered changes that propagated inland and eventually diverted the Colorado River through the growing Grand Canyon into the Gulf of California. The opening of the Gulf of California brought the ocean closer to the mountains, which steepened the streams flowing into the Gulf—the height of the mountains wasn't changed by opening the Gulf, but the horizontal distance a river had to flow from the mountains to sea level got shorter as the land ripped open.
In turn, this likely led to one of the rivers cutting into a high plateau and eventually cutting through a continental divide and diverting the ancestral Colorado River in an act of river piracy. A “continental divide” is the line on a map separating the rivers flowing to one ocean from the rivers flowing to another ocean, or somewhere else. As you might imagine (and as we discussed briefly back in the history of the closing of the proto-Atlantic and opening of the Atlantic in Module 4), the slope to one ocean from a continental divide is often steeper than the slope to the other ocean. The steeper side generally erodes faster, which causes the continental divide to move away from the steeper side toward the more-gradual side. (Eventually, this will lead to the slopes being similar on the two sides.)
But, the continental divide is irregular, not a straight line. Where a big river forms and cuts down, the slope from the divide to the river will be steeper than nearby, so erosion will be faster there and the divide will be forced away. Sometimes, this will cause the divide to intersect and “capture” the drainage of a stream that had been on the other side of the divide. (See the figure below.)
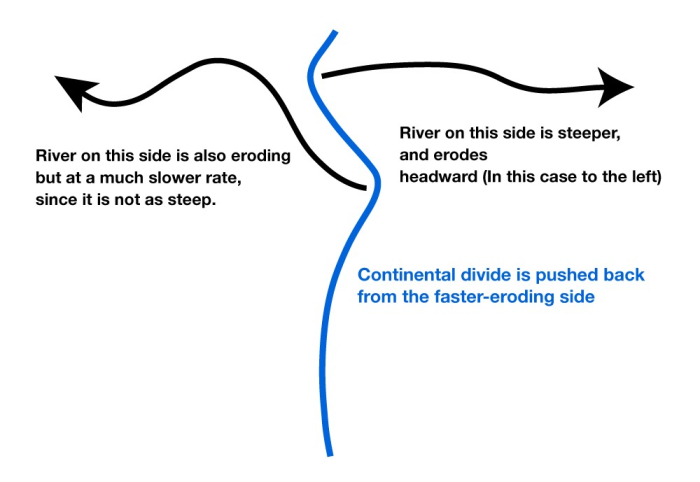
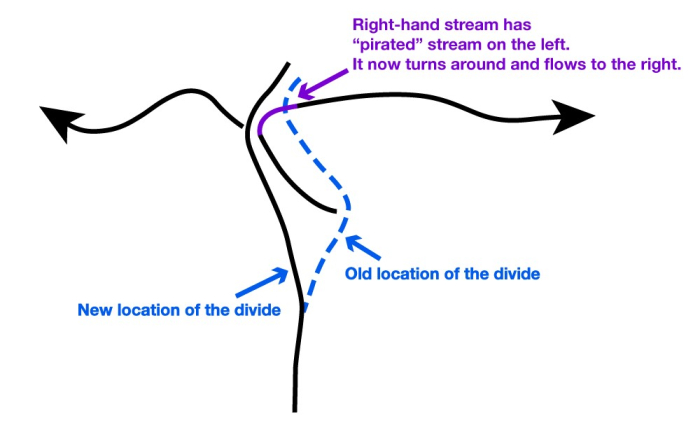
The upper panel in the figure shows two rivers (the black lines with arrows), separated by a continental divide (the blue line), viewed from above. If the right-hand river is steeper, then it will erode back until the headwaters of the left-hand river are “captured” in an act of “stream piracy,” as shown by the purple line.
There are lots of small rivers in the West with fairly big canyons—look at the most-of-a-mile deep canyon of the small Virgin River in Zion, or the remarkable amphitheater that tiny Bryce Creek has gnawed into the Paunsagunt Plateau to make Bryce Canyon. So, when the Gulf of California opened, the ancestral lower Colorado River steepened and cut headward (probably involving a piracy event with a stream exploiting the easily eroded San Andreas Fault), and pirated the ancestral upper Colorado River, which previously probably had drained internally (the river ran out into the desert and evaporated, the way some rivers do in Death Valley). This happened just over 5 million years ago; at that time, chunks of rocks of types that occur only up in the Rockies at the head of the Colorado River suddenly appeared in sediments of the Gulf of California, whereas before that time chunks of such rock types were absent in the Gulf of California.
You might think that with 5 million years of vigorous flow through the Canyon, the Colorado would have cut down even farther than it has, making an even deeper Canyon with a flatter, smoother river bed. But, the river has really had to cut the Canyon several times! Death-Valley-type Basin-and-Range faults associated with the opening of the Gulf of California also have cut across the Canyon, especially in the western end. Basaltic lava has come up some of the faults, in much the same way that the lava came up in Death Valley and in Red Canyon near Bryce. Several times over the last 5 million years, lava flows have dammed the Canyon, making lakes. Lakes accumulate sediment rather than eroding, while the erosive ability of the river is spent cutting through the dam. Once the river erodes the dam, it can then sweep out the loose sediment that accumulated in the lake, and then go back to deepening the Canyon… only to be dammed again by another lava flow. So, the river really had to cut down much more than a mile to make the canyon—cut down, get filled with lava, cut the lava, get filled again.
Why you don’t need to wait for a half-life to pass to measure it
In the text, you saw how radioactive decay occurs and provides “clocks” for the ages of rocks. Here, we go into a little more detail on the math, strictly for your entertainment and enjoyment.
The “law” of radioactive decay says that the more atoms of some radioactive parent you have, the more atoms of that parent will decay in some time. (There are many laws of this type: hotter bodies cool faster, rooms with more cats have more cats run out when you open the door, etc.). In addition, each radioactive parent type decays at its own particular rate, depending on the details of the quantum mechanics of its nuclear structure. Putting those words into math then goes like this. Given N parent atoms of some type, the change dN in the number of that type over some interval of elapsed time dt is:
The minus sign occurs because the number of parent atoms is decreasing over time as they decay to offspring. The K is a constant, called the decay constant (and often indicated with the Greek lambda, but we’ll stick with K). The numerical value of K is different for each different radioactive parent type, and includes the “physics” of how unstable the parent type is. A large K means a very unstable parent and a very rapid change to offspring; the units of K are inverse-time (so 1/seconds or 1/years).
If you never studied calculus, or you forgot what you studied, you won't make much sense of the next little bit. Don't worry. Those of you who took a calculus course and remember it will know that you can rearrange the equation to obtain:
Integrating yields: in which ln indicates the natural logarithm, C is a constant that we will determine, and t is the total time that has elapsed. Taking the exponential of both sides, and noting that the decay started at some time t=0 when there were parent atoms, yields the standard decay equation:in which exp indicates the exponential (it usually appears as ex or exp or inv ln on calculators). The negative in front of Kt is equivalent to writing . As t becomes large, becomes very large, so becomes very small—the equation says that after a long time, you run out of parent atoms, which is correct.
Notice that if you can measure , wait for some time and then measure N, the only unknown in this standard decay equation is K, so K can be calculated readily. The natural logarithm, ln, reverses the exponential so that . The natural logarithm appears on most calculators as ln or ln x or possibly as inv exp. Using this,
You usually will see this written as:
using one of the properties of logarithms.
We next estimate the half-life, . Note that after one half-life, . (So half of the parents have changed after one half-life.) If we let in the standard decay equation, take the natural logarithm of both sides, remember that , and rearrange, we obtain . This is the basis for the statement in the text that you do not need to wait for a full half-life to pass if you wish to learn the half-life; you just need to start with , wait for any time , measure N, calculate K from this, and then calculate from K. The half-life is useful, but most professionals in the field use the decay constant K most of the time because K is more “fundamental” (it appears in the statement of decay given first above, and does not need to be derived as for the half-life).
Radiocarbon Revisited
You won’t have to look very far on the web to find sites—usually attached to certain religious ideas—complaining about errors in radiometric dating. (And Dr. Alley was once shown a published tract pointing out how stupid Dr. Alley himself must be to think that he could count more annual layers in an ice core than the total age of the Earth as estimated from writings in a particular religious text!) Some of the objections to radiometric dating are fairly silly, and even some of the young-Earth sites have put up notes asking followers to avoid using certain common arguments against scientists because those arguments are just wrong. The “5000-year-old” living clam falls in this category, as described later in this enrichment. The bottom line is that radiometric dating is useful, practical, successful, matches written records as far back as they go, matches other indications beyond that, and reveals a deep and fascinating history. Radiometric dating is not perfect, it does include errors, and practitioners have to know what they’re doing and think about it, but it works.
Skeptics about the use of scientific age dating in geology and the age of the Earth have especially focused on complaining about radiocarbon dating. This focus is odd, because radiocarbon—also called carbon-14—is not used in establishing the age of the Earth, or the age of the main geological events. The half-life of radiocarbon is only 5730 years; samples older than about 50,000 years have nearly run out of radiocarbon and so cannot be dated by radiocarbon. But, radiocarbon is used a lot in dating archaeological sites, and this may have caught the attention of people who study early written histories. In addition, as you will see, radiocarbon is more complex than many others (such as the potassium-argon system discussed in the regular text), and it may be easier to argue about complex things.
Much of the complexity of radiocarbon arises because the offspring of radiocarbon (the gas nitrogen-14) is very common, and is not retained well by the samples that are dated using radiocarbon (wood, charcoal, bone, or other formerly living things—not most rocks). Thus, radiocarbon dating does not look at the parent-to-offspring ratio; instead, the starting concentration of radiocarbon is estimated, the concentration today is measured, and the ratio gives the age. Radiocarbon is mostly made in the atmosphere, when cosmic rays collide with atoms and knock off neutrons that then hit nitrogen-14 nuclei and make carbon-14. This doesn’t happen very rapidly; natural production is just about 15 pounds for the whole Earth per year, or just over two carbon-14 atoms per square centimeter (just under 1/2 inch on a side) of the Earth’s surface per second.
In the atmosphere, radiocarbon quickly combines with oxygen to make carbon dioxide. The atmosphere is well-mixed—release some gas molecules here, and within a few years they will be spread fairly uniformly around the planet—so the radiocarbon-bearing carbon dioxide is quite uniformly distributed around the globe. Green plants grow by using carbon dioxide, and roughly one of each trillion carbon atoms in the atmosphere and in green plants is carbon-14 rather than stable types of carbon-12 or carbon-13. Plants are eaten by animals. Most animals live less than 100 years, whereas most carbon-14 lasts thousands of years, so when plants and animals die, they have just about the same ratio of carbon-14 to carbon-12 as was in the atmosphere when they were still alive. After plants or animals die, they do not breathe or eat any more, so they don’t take in carbon-14 while the carbon-14 in them decays. Hence, the ratio of carbon-14 to carbon-12 in a formerly living material is a clock.
Whole textbooks can be written refining the previous two paragraphs, and a scientific journal, Radiocarbon, focuses almost exclusively on the topic. If you aren’t a real stickler for accuracy—if “this died sometime between 9,000 and 11,000 years ago” is good enough for you—then you really don’t need a whole journal devoted to radiocarbon. (You still need to worry about one or two things that we’ll come to, but not about too many.) But if you want to get the answer right to within a few decades or less, then you have to be really careful.
One problem is that production rates of radiocarbon have varied over time. When the sun is more active or the Earth’s magnetic field is stronger, they protect us more from cosmic rays and reduce production of radiocarbon. The changes are not huge, and there are ways to correct for them (changes in the strength of magnetization can be estimated by measuring the degree of alignment of the “magnets” in lava flows or sediments of different ages, and the activity of the sun can be tracked from the magnetic measurements plus the ice-core concentrations of beryllium-10, which is also made by cosmic rays).
Changes in the Earth’s carbon cycle also matter a little to the history of the starting concentration of carbon-14 in plants and animals in the past. For example, now we are pulling up immense quantities of really old fossil fuels that do not have any remaining carbon-14, and burning those fossil fuels to make carbon-14-free carbon dioxide that goes into the atmosphere, diluting the carbon-14 there. When we humans were busily blowing up atomic bombs in the atmosphere, they made a lot of carbon-14. Before we were so influential, changes in carbon-14 in the atmosphere were MUCH smaller, and changes in ocean circulation were probably most important—some carbon dioxide goes from atmosphere to ocean, and the ocean waters sink in certain places and spend a thousand years or so down deep before coming back up to exchange carbon dioxide with the atmosphere. Because some of the carbon-14 from the atmosphere ends up decaying in the deep ocean, the ocean circulation actually reduces atmospheric radiocarbon—if water didn’t sink into the deep ocean, there would be less carbon-14 there and less carbon-14 decay there, and that would leave more carbon-14 in the atmosphere. At certain times in the past, less sinking of ocean waters seems to have occurred, allowing more carbon-14 to exist in the air.
The usual way to handle all of this is to use radiocarbon to date tree rings (which quit exchanging carbon with the atmosphere as soon as they grow) or shells in annually layered sediments, and use the layer-counted ages and the known half-life of radiocarbon to calculate the starting concentration of radiocarbon. Because radiocarbon is well-mixed in the atmosphere, and must have been well-mixed in the past, a calibration curve developed from samples anywhere on Earth can be used for samples from anywhere else. You can also date some samples, such as corals or cave formations, using two techniques: an accurate technique such as uranium-series disequilibrium, and radiocarbon, and so obtain a calibration curve for the radiocarbon. Many different calibration studies have been conducted, and while they do not agree perfectly and research is ongoing, they agree reassuringly well. The biggest corrections are a bit more than 10% with uncertainties of less than 1%—a sample that looks to be 10,000 years old, assuming that there were no changes in radiocarbon concentration of the atmosphere, is actually about 11,500 years old, because the radiocarbon concentration of the atmosphere did change, and the uncertainty in this is less than 100 years.
If you are primarily interested in the question “Does the world really look older than written records”, even radiocarbon provides a very good answer (“Yes, with very high scientific confidence”). Science has long since moved past that question, and the research frontier involves numerous fascinating questions, such as whether we can reconstruct changes in ocean circulation from the changing calibration of the radiocarbon clock after correcting for the changes in the sun and the magnetic field.
Plants actually have a slight preference for carbon-12 over carbon-13 or carbon-14 (the lighter atoms diffuse into the plant and react more easily), so the concentration of carbon-14 in a plant is slightly less than the concentration in the air. The preference for carbon-12 over carbon-13 is half as big as the preference for carbon-12 over carbon-14, so measuring the concentrations of all three types allows an accurate correction; this measurement is made easily and is done routinely when highly accurate dates are needed, so it should not bother anyone much.
The 5000-year-old living clam raises a different but interesting issue. All of the discussion so far has assumed that the items being dated obtained their carbon from the atmosphere. That is almost always a pretty good approximation for almost everything. But suppose that you “ate” only things that had been dead for a long time—you would not take in much radiocarbon, and so you would look old to someone who assumed that you ate things containing normal concentrations of radiocarbon. Certain special ecosystems on the sea floor do just that; they live on natural oil seeps, eat the oil or eat things that ate the oil, and the oil is old and so lacks radiocarbon. If you were stupid enough to sample these and assume that they were eating “normal” foods, then you would mistakenly assume that the living creatures had been dead for a long time.
Such oil-seep ecosystems are quite rare and special. A more-common situation is a clam in a creek in a carbonate terrain. When caves are being made, the chemical equation for the water and carbon dioxide dissolving the rock is:
The rain and atmospheric carbon dioxide on the left of the equation combine with the calcium carbonate of the limestone, yielding the calcium and bicarbonate ions on the right-hand side of the equation that are freed to wash down the creek. If a clam is making its shell from the water, the clam just runs this reaction backward. Notice, however, that half of the carbon, C, in the water came from the atmospheric and half from the rock. The rock is almost always very old, and has no radiocarbon. So, a clam in this situation would form a shell with only half as much radiocarbon as for a clam growing in a stream that does not drain carbonate rocks and that gets all of its carbon from the atmosphere. Hence, if scientists were clever with their instruments but stupid otherwise, those scientists might end up thinking that a living clam had been dead for over 5000 years.
Scientists are fully aware of this. For decades, however, there was a convention of reporting all radiocarbon measurements as the equivalent age assuming that the sample had been in equilibrium with the atmosphere. Dr. Alley is reasonably confident that the myth of the clam that was living yet the scientists thought it was thousands of years old came from work by a distinguished senior colleague, who in the 1960's published papers listing dates in the conventional fashion. That colleague actually was using the results to learn about the geochemistry of the waters. As noted above, some of the young-Earth-creationist websites have asked their supporters to “clam up” about this, because using it in an attempt to discredit scientists instead makes the young-Earth-creationists look confused.
As a possibly interesting aside, the natural flavoring vanilla is obtained from the pods of a tropical orchid, but the main chemical in vanilla can also be obtained from petroleum much more cheaply. This creates an incentive for cheaters to sell petroleum-extracted vanilla as the real thing. Cheaters can be caught, though, because real vanilla contains radiocarbon but the fossil-fuel version does not. When Dr. Alley was writing this, commercial testing for a small fee was available to protect consumers and natural-vanilla producers.